كم مرة ينطبق عقربا الساعة على بعضهما في اليوم الواحد ؟
How many times do a clock's hands overlap in a day ?
In order to solve this question, we are going to define the equation of each Needle.
The equations of the 3 Needles are based on their respective rotation, and we get the following 3 equations:
The equations of the 3 Needles are based on their respective rotation, and we get the following 3 equations:
- Position of the hour’s Needle is equal to:
- Position of the Minute’s Needle is equal to:
- Position of the Second’s Needle is equal to:
30°.h+v(h)≔30°.h+[30/60°.m+30/3600°.s]≔30°.h+[1/2°.m+1/120°.s]
6°.m+v(m)≔6°.m+6/60.s≔6°.m+0,1°.s
6°.s
Now, we are going to solve the equations pairwise by setting them equal.
Equation (2) and (3) gives us that the position of the Minute’s Needle is equal to the Second’s Needle:
Equation (2) = Equation (3)
6°.m + 0,1°.s = 6°.s
→ m = (5,9°/6°) . s
Equation (1) and (2) gives us that the position of the Hour’s Needle is equal to the Minute’s Needle:
Equation (1) = Equation (2)
30°.h + 0,5°.m + 1/120°.s = 6°.m + 0,1°.s
30°.h = 5,5°.m + 11°/120°.s
We now set the value of ‘m’ found on the first equality:
30°.h = 5,5°.(5,9°/6°).s + (11°/120°).s
30°.h = [(32,45°/6°)+(11°/120°)].s = 660/120.s = 11/2.s = 5,5.s
→ s = (30/5,5) . h
And, we know that :
m = (5,9/6) . s
→ m = (5,9/6) . (30/5,5) . h = (5,9/5,5) . 5 . h = 5,36…36 h
Let’s be the hour 6 o’clock, this means that:
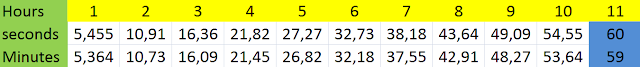
s = (30/5,5) . 6 ≈ 32,7272
m = (5,9/5,5) . 5 . 6 ≈ 32,18
Because ‘m’ must be a natural number, the overlapping is not possible at that time
Now, let’s be the hour 1 o’clock then we have:
s = (30/5,5) . 1 ≈ 5,45
m = (5,9/5,5) . 5 . 1 ≈ 5,36
Because 'm' is a natural number, the overlapping is not possible. Analog, we can disprove the rest of the value except for 11 o’clock.
When the hour is 11 o’clock then we have:
s = (30°/5,5°) . 11 = 60
m = (5,9°/5,5°) . 5 . 11 = 59
That means that the hour is actually 11h 59mn 60s which means 12 o’clock.
Congratulation, we have just found the hour when all needles are overlapping. The complete overlapping is only possible at exactly 12h 0mn 0s!
Here is the results for all hours: